Wave created by LC circuit
As we know, the basic physic model of oscillatory discharge is an LC circuit, whose resonance frequency is given by 
. Therefore by varying the length of wire loop used for discharge, we will be able to get waves of different frequency. Here we use single wire loops with length of 1m, 2m, 3m and 4m to discharge the same Leyden jar (the parameter of this Leyden jar can be found here).
The inductance of the wire loop can be calculated by 
, or online calculator.
Following is the calculated results and measured results. The resonance frequency is then calculated using C=1800pF:
| Loop size: | Calculated value(H) | Freq=1/(2*PI*SQRT(LC)) (Hz) |
| 1m, R~=50cm, d=1mm | 3.95467E-06 | 1886378.609 |
| 2m, R~=60cm, d=1mm | 4.88307E-06 | 1697608.166 |
| 2m, R~=90cm, d=1mm | 7.78317E-06 | 1344638.442 |
| 3m, R~=120cm, d=1mm | 1.08114E-05 | 1140888.843 |
The setup is shown below:
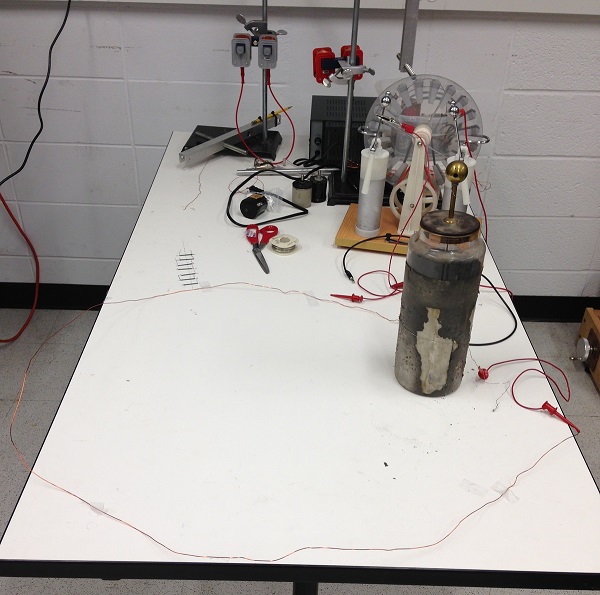
Wave detection
The wave is detected using an oscilloscope with two short wires attached which function as an antenna:
After the wave was captured by the oscilloscope, it was then saved to a USB and more analysis could be done using computer.
Results
Below is a summary of the results. According to the equation for LC circuit, the theoretical ratio between two frequency equals to the square root of the ration of wire length. Click the links to view the waveforms and frequency analysis generated by different wire loops.
|
length |
Frequency(Hz) | Avg. Freq.(Hz) | Ratio |
Theoretical ratio |
| 1 | 1996805 | 1m / 2m: 1.240487734 | ||
| 1 | 2158556 | 2077680.5 | 2m / 4m: 1.319149986 | 1:2==1.414213562 |
| 2 | 1597444 | 2m / 3m: 1.157499627 | 2:3==1.224744871 | |
| 2 | 1752336 | 1674890 | 3m / 4m: 1.139654783 | 3:4==1.154700538 |
| 3 | 1484561 | |||
| 3 | 1400000 | |||
| 3 | 1456408 | 1446989.667 | ||
| 4 | 1366950 | |||
| 4 | 1214575 | |||
| 4 | 1227496 | 1269673.667 |
